Multiscale models for predicting the thermal and mechanical behaviour of masonry

1. Introduction

As a contribution to lowering global CO₂ emissions, reducing heat losses of our buildings has been a driving force for innovations in the clay brick and tile industry for decades. Such a reduction of the heat flow can be achieved with additional pore volume on two levels of observation: on the level of the clay brick with the development of vertically perforated brick geometries that have been optimized with respect to their thermal insulation properties, and on the level of the clay brick body with the addition of suitable pore-forming agents. Advanced developments in recent decades, however, have been based predominantly on the empirical knowledge gathered by experienced employees rather than on physically based modelling concepts. The development of such concepts on the basis of innovative analytical methods, combined with numerical simulation tools for calculation of the properties of brick masonry based on microstructural characteristics was therefore principal objective of the research project.

The effective (macroscopic) properties of the brick body have been observed on different hierarchical structural levels as a function of the hierarchical structure. While on the highest level, macropores are still visible to the naked eye (these are formed, for example, with the addition of EPS or sawdust), the heterogeneous composition on smaller length scales (lower analysis levels) can only be observed with a scanning electron microscope. Micropores are distributed throughout a clay matrix that contains amorphous constituents and classical clay minerals as well as relatively large pores. These different levels of observation necessitate the application of multiscale models to model the dependence of the physical properties of the brick body on the microstructure. To this end, meaningful observation levels for the brick body are identified, and the properties of the material phases (morphology, thermal and mechanical properties) are determined on each of these observation levels. Depending on the latter, analytical homogenization processes can then be applied to determine the macroscopic properties of the brick body. With the help of these calculation methods, variation studies are possible, which permit an identification of key parameters affecting the macroscopic properties.

For the calculation of effective properties of the masonry, numerical simulation tools can then be applied, which enable the prediction of the load-bearing capacity of brick in brickwork [Kiefer 2017]. With these models, which enable the calculation of effective properties on the basis of microstructural characteristics, a useful tool is therefore available for optimization of the brick body on the basis of physically founded models.

2. Identifying the observation scales

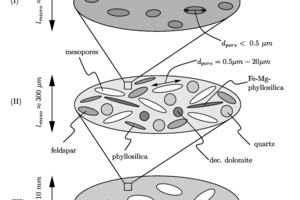
The multiscale models mentioned in the introduction enable the homogenization of material properties over several observation scales. A fired brick body is perceived by the observer as a (relatively) homogeneous material, if, however, this (macroscopically) homogeneous material is analysed on different length scales, the constituent material phases (for example, quartz, mica and clay minerals) become visible. Correlating the physical properties of these material phases (like thermal conductivity, stiffness and morphometry) with macroscopic material properties is the objective of multiscale analysis and necessitates the definition of length scales, for which the following apply: d « l « L, where d represents the characteristic length of the material phases, I the characteristic dimension of the length scales, respectively the representative volume unit (RVU), and L the characteristic length of the load on the RVU. With these preconditions, three observation levels can be identified for brick bodies:

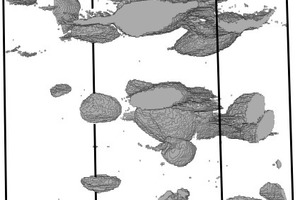
Macrostructure: This comprises relatively large pores (up to several millimetres) in the brick body, which are formed as a result of the addition of coarse poreformers (»Fig. 1), namely expanded polystyrene (EPS) and sawdust (SD) These are visible to the naked eye. We refer to this observation level as the macroscale, consisting of a clay matrix that contains macropores. The morphology of this pore volume can be determined by means of computer tomography [Kariem 2017]. With use of the non-commercial software ImageJ REF, these pores are approximated geometrically as ellipsoids, as a result of which solid angle, volume percentage and slenderness (ratio of the semi-axes of the ellipsoids) of every individual pore can be acquired.

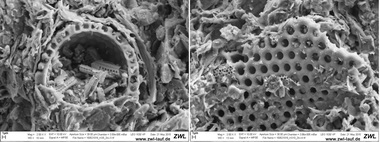
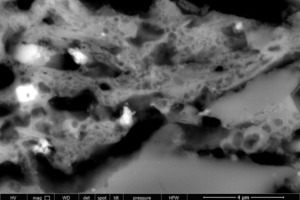
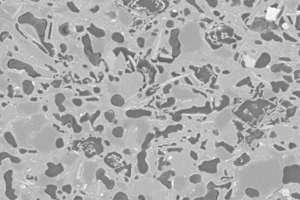
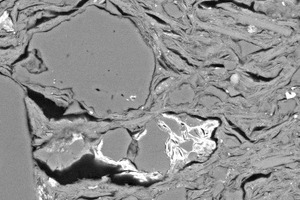
Mesostructure: This describes the clay matrix observed macroscopically as homogeneous, which is now resolved in more detail as a result (»Fig. 2). The combination of a scanning electron microscope with an energy-dispersive X-ray spectroscope allows accurate determination of the phases present [Kariem 2020b]. Like the process on macroscale, the individual phases are captured as ellipsoids with the software ImageJ REF. Additional pore volume is formed with sawdust and paper sludge (PS).

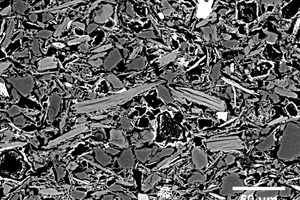
Microstructure: This describes the matrix identified on the mesoscale, which contains further micropores that can be identified to some extent but cannot be fully resolved with optical methods (»Fig. 3). For this reason mercury porosimetry measurements were conducted on the test specimens to determine the microporosity in the matrix. The identified pores are assigned the same morphometric properties as those on the mesolevel, as an optical capture of the solid angle and slenderness of the pores is not possible on this length scale. Paper sludge residue induces additional pores on this observation level.

3.Identifying the material phases

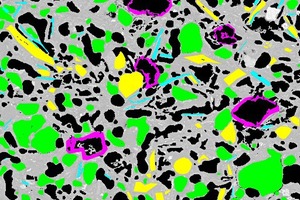
For the determination of the material phases on mesolevel, a multistage process is required: in a first step, the mineralogical composition of the test specimens is analysed by means of X-ray diffractometry oder cristallography (XRD). With an electron microscope (SEM), structures can then be identified on the observed length scale. Combination of the structural analysis of the SEM with the results of the energy-dispersive X-ray spectroscopy (EDX) measurements enables assignment of the structures to the minerals previously determined by means of XRD (»Fig. 4). This process was applied on brick specimens with different chemical composition (Clay HD is rich in carbonates, Clay KV, on the other hand, is low in carbonates while it has a high content of quartz) and firing temperature (fired at 880 °C and 1 100 °C). A verification of the results is possible based on accompanying X-ray fluorescence measurements (XRF) and a qualitative analysis of the development of the material phases with increasing firing temperature. In addition, determination of the chemical composition by means of XRF allows, in combination with the XRD results, determination of the chemical composition of the clay matrix and its development as a function of the firing temperature.

Moreover, the SEM images enable the determination of the morphometric properties of the material phases. Captured here are the orientation, the volume percentage and the slenderness of the structures approximated with ellipses.

4. Identifying the thermal and mechanical properties of the material phases

The volume percentage, the orientation and the slenderness of the material phases are known thanks to the above-described methods on every identified length scale. For homogenization of material properties, the physical properties (thermal conductivity and stiffness) are necessary. Scanning Thermal Microscopy (SThM) is used for determination of the thermal conductivity [Kiefer 2020] and nanoindentation (NI) [Kariem 2020] is used for determination of the stiffness.

4.1.Determining the thermal conductivity by means of scanning thermal microscopy

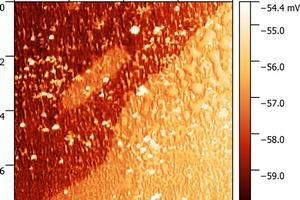
Literature references on the thermal conductivities of the individual phases cannot be applied as these show a very wide scatter depending on the exact chemical composition and purity of the material. It is therefore essential to determine the thermal conductivities of the individual phases of the studied clay. One possibility for this is scanning thermal microscopy, the principle of operation of which can be outlined as follows: A V-shaped wire with constant temperature (typically about 160 °C) is moved over the surface of the specimen while the energy necessary to keep the temperature in the wire constant is measured. This can be determined based on measurement of the temperature-dependent resistance in the wire. To quantitatively determine the thermal conductivity of the specimen, calibration based on a previously defined standard is necessary as the heat flow between specimen and wire depends on several parameters (for instance the contact radius, the heat loss caused by convection, near-field radiation or the transfer of heat between specimen and wire by a liquid film [Majumdar 1999]). To determine the thermal conductivity, the following correlation between thermal conductivity and necessary heat energy was used [Fischer 2005]:

where ΔQ denotes the necessary heat flow through the wire, A and B represent calibration parameters, and λ_g the averaged thermal conductivity, which is formed as follows:

with λ_s as the thermal conductivity of the specimen and λ_g as the thermal conductivity of the tip of the SThM. The two calibration parameters were determined with the help of four calibration materials with known thermal conductivity (polymethyl acrylate, synthetic quartz glass, corundum and copper). All specimens and calibration materials were cast in epoxide resin and ground according to the same polishing protocol to achieve a constant roughness. This was measured by means of AFM as being between 2 and 20 nm. According to [Fischer 2005], reliable measurements can be obtained for specimens with a roughness of the specimen surface up to 10 nm at low thermal conductivities (< 20 W/mK). After the tip of the SThM similar to an atomic force microscope (AFM) has captured the morphology of the surface, measurements with high roughness can be identified and excluded from the evaluation [Kiefer2020].

4.2. Determining stiffness by means of nanoindentation

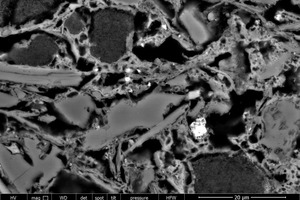
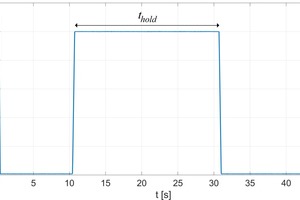
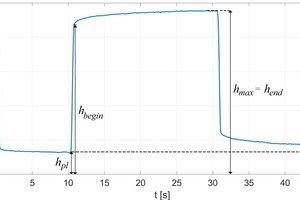
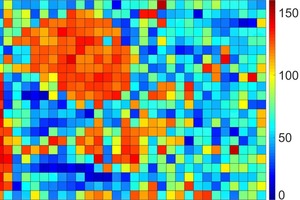
For the determination of the mechanical properties of the material phases, extensive measurements were conducted by means of nanoindentation [Kariem 2020a]. The stiffness of the material phases, together with the determined morphometric parameters, then serves as an input value for the homogenization. With the classification of material phases by means of EDX, nanoindentation measurements performed in a defined pattern (on an area measuring 96 μm x 66 μm at a spacing of 3 μm) can be assigned to the different phases (»Fig.7). To enable the determination of the elastic part of the material response (particular phases exhibit pronounced viscoelastic material behaviour), the load curve consists of several load and load-release phases: the first load and load release phase takes place with the highest technically possible velocity with a maximum load of 280 μN, to pre-empt plastic deformations. The almost full load release before the second load and load-release phase with a duration of ten seconds and very low load (2 μN) enables the recovery of viscoelastic deformations. The second load of 250 μN is applied within 0.3 seconds, held 20 seconds, and released again completely within 0.3 seconds. The very short load and load-release time is necessary to minimize the influence of viscoelastic material behaviour on the material response. A 15-second phase with another very small load (2 μN) completes the load function (»Fig. 6).

The part of the elastic properties can be determined from the load relief of the second load and load-relief phase. To enable assignment to the individual material phases and to avoid influences of the surrounding material on the measurement as far as possible, here a very small indentation depth of around 50 nm is chosen. This requires, like the SThM-measurements, a very low roughness of the specimen surface. A total number of around 8 000 indents guarantees a statistically significant statement on the stiffness of the individual material phases.

5. Multiscale model for homogenization of the

material properties

The multiscale model for homogenization of the physical properties of the brick body is shown in »Fig. 8, the individual RVUs correspond to the observation levels identified in Chapter 2. The orientation of the individual material phases is taken into account by means of an orientation distribution function [Ulm 2005], so that the morphometric properties can be considered in their entire complexity. Homogenization on the basis of the individual RVUs is then performed by means of a Mori-Tanaka scheme [Mori 1973], as the individual material phases are mostly enclosed in a matrix. The microlevel of the model forms a matrix consisting of clay mineral and amorphous constituents, which becomes increasingly vitrified with higher firing temperature. Embedded in this are micropores with a diameter < 0.5 µm. The observation level next in size is the mesoscale at which the material phases and mesopores identified by means of SEM-EDX are enclosed by the matrix homogenized in Step 1. The homogenized mesolevel in turn forms the matrix on macrolevel, which includes pores and cracks.

6. Verification of the multiscale model

6.1. Determining the thermal conductivity of the brick body

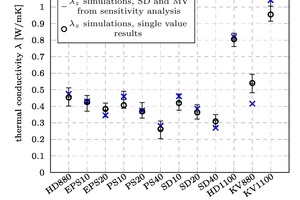
The thermal conductivity of the brick body was determined on twelve different test specimens by means of a heat flow meter (on the two basic clays HD/KV, fired at each 880 °C and 1 100 °C, as well as specimen HD880 with different volume percentages of poreformers). Here specimens were successively ground down to enable calculation of the influence of the heat transmission resistance from the resulting multi-thickness measurements. A comparison between the values predicted with the multi-scale model as a function of the determined variance of the input parameters with the results of the heat flow meter shows very good agreement (»Fig. 9). Additional studies show a distinct dependence of the thermal conductivities obtained on three key parameters: the porosity on micro- and mesolevel, as well as the thermal conductivity of the matrix consisting of clay minerals and amorphous constituents.

6.2. Determining of the stiffness of the brick body

The stiffness of the brick body was determined with ultrasound measurements on account of the small sizes of the specimen. Measurement was performed by means of a pulse transmitter, which consists of a pulse receiver, an oscilloscope and two ultrasonic signal generators. The signal generators or transducers contain piezoelectric elements that as pulse transmitters transform the electric signal into a mechanical signal, and vice versa in the case of the pulse receiver. Then, from the measured time needed by the ultrasonic wave to penetrate the specimen and the thickness of the specimen, a conclusion can be made with regard to the velocity of the ultrasound:

For the case of longitudinal waves, to which the experiments described here are limited, a direct correlation results between the measured velocity of the ultrasonic wave and the normal components of the stiffness tensor (theory of plane waves in a continuum [Carcione 2015]):

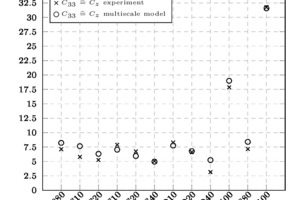
If the results of the multiscale modelling are compared with the measured results of the ultrasound method, then good agreement is also found for the homogenization of the mechanical properties (»Fig. 10).

7 .Summary and outlook

With the multiscale model presented, for the first time, physical properties of the brick body can be predicted as a function of the chemical and mineralogical composition and added poreformer. This provides a time- and cost-efficient possibility to enhance the performance of the brick. In addition, the multiscale model allows specific studies to evaluate individual input parameters, like, for example, the effectiveness of additional pore volume on different observation scales. In an ongoing research project (“Innovative Brick 2”) conducted by Vienna University of Technology and Wienerberger AG, other clays and poreformers characteristic for production of bricks in the EU are being studied. Moreover, initial findings concerning extension of the multiscale model to predict the strength are promising.